
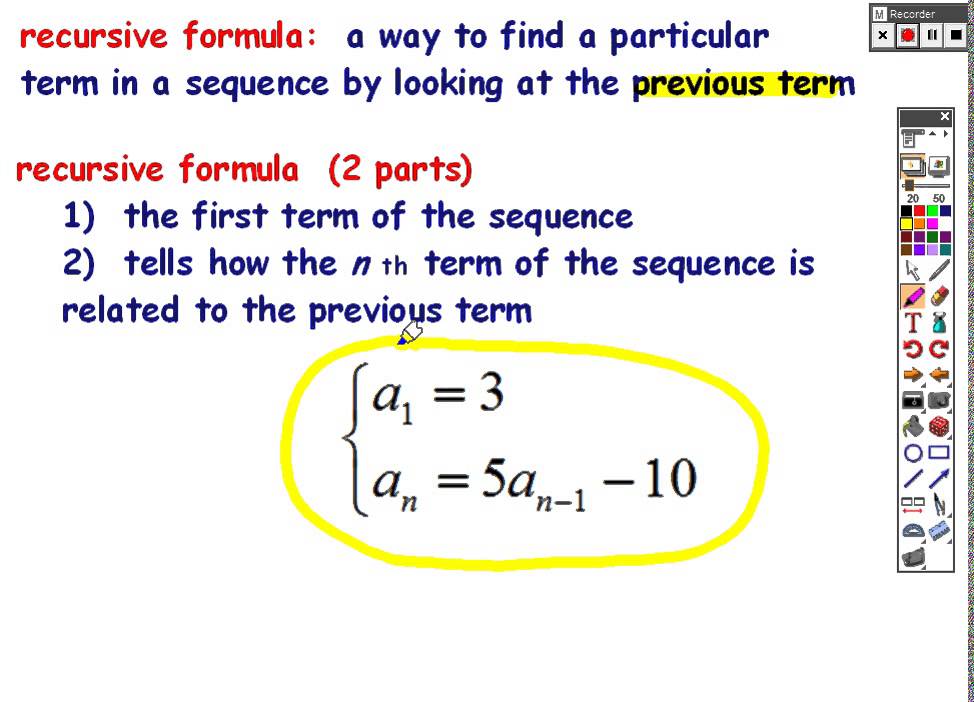
Maybe these having two levels of numbers to calculate the current number would imply that it would be some kind of quadratic function just as if I only had 1 level, it would be linear which is easier to calculate by hand. Arithmetic Sequence Recursive formula may list the first two or more terms as starting values depending upon the nature of the sequence.
Recursive formula for arithmetic sequence how to#
This gives us any number we want in the series. In this video you will learn how to write a recursive formula or rule for an arithmetic sequence, how to use sequence notation to write the terms of an arithmetic sequence, and given a. Step 3: Develop the answer of your subproblem, and use it to determine the original problem. Step 2: Find out subproblem from function and assume your function already works on it. I do not know any good way to find out what the quadratic might be without doing a quadratic regression in the calculator, in the TI series, this is known as STAT, so plugging the original numbers in, I ended with the equation:į(x) = 17.5x^2 - 27.5x + 15. Step 1: First of all, get a clarified idea about what your function should do. Then the second difference (60 - 25 = 35, 95-60 = 35, 130-95=35, 165-130 = 35) gives a second common difference, so we know that it is quadratic. = a ( 4 ) + 2 =a(4)+2 = a ( 4 ) + 2 equals, a, left parenthesis, 4, right parenthesis, plus, 2 = 9 =\goldD9 = 9 equals, start color #e07d10, 9, end color #e07d10Ī ( 5 ) a(5) a ( 5 ) a, left parenthesis, 5, right parenthesis = 7 + 2 =\blueD 7+2 = 7 + 2 equals, start color #11accd, 7, end color #11accd, plus, 2 = a ( 3 ) + 2 =a(3)+2 = a ( 3 ) + 2 equals, a, left parenthesis, 3, right parenthesis, plus, 2 = 7 =\blueD 7 = 7 equals, start color #11accd, 7, end color #11accdĪ ( 4 ) a(4) a ( 4 ) a, left parenthesis, 4, right parenthesis = 5 + 2 =\purpleC5+2 = 5 + 2 equals, start color #aa87ff, 5, end color #aa87ff, plus, 2 = a ( 2 ) + 2 =a(2)+2 = a ( 2 ) + 2 equals, a, left parenthesis, 2, right parenthesis, plus, 2
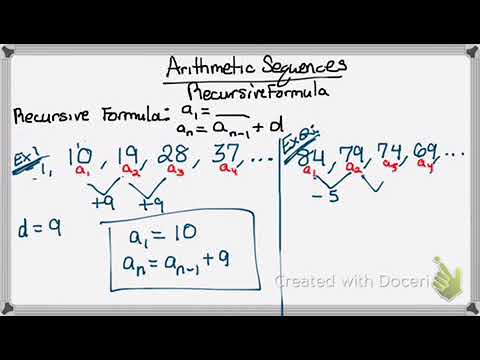
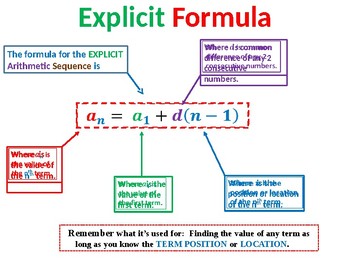
= 5 =\purpleC5 = 5 equals, start color #aa87ff, 5, end color #aa87ffĪ ( 3 ) a(3) a ( 3 ) a, left parenthesis, 3, right parenthesis = a ( 1 ) + 2 =a(1)+2 = a ( 1 ) + 2 equals, a, left parenthesis, 1, right parenthesis, plus, 2 = 3 =\greenE 3 = 3 equals, start color #0d923f, 3, end color #0d923fĪ ( 2 ) a(2) a ( 2 ) a, left parenthesis, 2, right parenthesis = a ( n − 1 ) + 2 =a(n\!-\!\!1)+2 = a ( n − 1 ) + 2 equals, a, left parenthesis, n, minus, 1, right parenthesis, plus, 2Ī ( 1 ) a(1) a ( 1 ) a, left parenthesis, 1, right parenthesis To summarize the process of writing a recursive formula for an arithmetic sequence: 1.
A ( n ) a(n) a ( n ) a, left parenthesis, n, right parenthesis


 0 kommentar(er)
0 kommentar(er)
